Mathematicianadda

submitted by /u/Brouhoff
[link] [comments]

from math https://ift.tt/2HNxoZ6

Reducing Rational Expressions to Lowest Terms Worksheet

from Math Blog https://ift.tt/2uDlWGV

How to Find the Excluded Value of a Rational Expression

from Math Blog https://ift.tt/2WBCRWe

Let $(\bar{M},\bar{g})$ and $(\dot{M},\dot{g})$ be two Riemannian manifolds and let $f\in C^{\infty}(\bar{M})$ be nowhere zero. Let $(M^n,g)$ be the warped product of the two manifolds with warping function $f$; that is, $M=\bar{M}\times\dot{M}$ and \begin{equation} g=\bar{g}\times_f\dot{g}:=\bar{\pi}^*\bar{g}+(f\circ\bar{\pi})^2\dot{\pi}^*\dot{g} \end{equation} where $\bar{\pi}:\bar{M}\times\dot{M}\to\bar{M}$ and $\dot{\pi}:\bar{M}\times\dot{M}\to\dot{M}$ are the natural projections. (If you like, we can simply write $g=\bar{g}+f^2\dot{g}$).

Convention on indices: $1\leq a,b,c,\cdots\leq q$ for $(\bar{M},\bar{g})$, $q+1\leq\alpha,\beta,\gamma,\cdots\leq n$ for $(\dot{M},\dot{g})$ and $1\leq i,j,k,\cdots\leq n$ for $(M,g)$. Einstein summation convention is assumed.

Let $\left\{\bar{\omega}^a\right\}_{a=1}^q$ and $\left\{\dot{\omega}^{\alpha}\right\}_{\alpha=q+1}^n$ be local orthonormal coframes on $(\bar{M},\bar{g})$ and $(\dot{M},\dot{g})$ respectively. Then a local orthonormal coframe on $(M,g)$ can be given by \begin{align} \omega^i:=\left\{ \begin{array}{ccl} \bar{\pi}^*\bar{\omega}^i & \mbox{if} & 1\leq i\leq q \\ (f\circ\bar{\pi})\dot{\pi}^*\dot{\omega}^i & \mbox{if} & q+1\leq i\leq n \end{array} \right. \end{align} (Again, if you like, we can simply write $\bar{\omega}^i$ and $f\dot{\omega}^i$ respectively). Moreover, under these two coframes, denote the connection 1-forms by $\bar{\omega}^b_a$ and $\dot{\omega}^{\beta}_{\alpha}$ respectively.

My goal is to compute the connection 1-forms of $(M,g)$. By taking exterior derivative of the definition of $\omega^i$, applying the Cartan's 1st structural equation and gather the terms to the LHS, I arrive at \begin{gather} \omega^b\wedge\big(\omega^a_b-\bar{\pi}^*\bar{\omega}^a_b\big)+\omega^{\beta}\wedge\omega^a_{\beta}=0 \\ \omega^b\wedge\left(\omega^{\alpha}_b-\frac{(f\circ\bar{\pi})_b}{f\circ\bar{\pi}}\omega^{\alpha}\right)+\omega^{\beta}\wedge\big(\omega^{\alpha}_{\beta}-\dot{\pi}^*\dot{\omega}^{\alpha}_{\beta}\big)=0 \end{gather} where $(f\circ\bar{\pi})_i$ is defined via $d(f\circ\bar{\pi})=(f\circ\bar{\pi})_i\omega^i$.

Now it is tempting to conclude directly from above that \begin{align} \omega^a_b&=\bar{\pi}^*\bar{\omega}^a_b \\ \omega^{\alpha}_{\beta}&=\dot{\pi}^*\dot{\omega}^{\alpha}_{\beta} \\ \omega^{\alpha}_b&=\frac{(f\circ\bar{\pi})_b}{f\circ\bar{\pi}}\omega^{\alpha} \end{align} but I don't think this is valid in general for sum of wedge products. The Cartan's lemma tells us that at most we only can conclude that the expressions in the parentheses can be written as a linear combination of the $\omega^i$'s (this is trivial here) with coefficients satisfying some symmetry on the indices.

Hence, I would like to ask for the way to proceed. How do we continue to compute the connection 1-forms? Any comment, hint and answer is welcomed and appreciated.

P.S. This is not a homework problem. I was just trying to explore and play around the things on my own. Thus, there is a chance that what I have written contains some errors. Feel free to correct me if I have written something wrong.

from Hot Weekly Questions - Mathematics Stack Exchange

I'm trying to prove $$\ln k \geq \int_{k-\frac{1}{2}}^{k+ \frac{1}{2}}\ln x dx$$

In other words, I'm trying to show why the area of the rectangle with height $\ln k$ and width $1$ bounds the area under the graph of $f(x)=\ln x$ in the interval $[k-\frac{1}{2},k+\frac{1}{2}].$

I tried to integrate but got stuck. Any ideas for an elegant proof for this?

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/RepublicanRandy
[link] [comments]

from math https://ift.tt/2uDN88E

Let consider a square $10\times 10$ and write in the every unit square the numbers from $1$ to $100$ such that every two consecutive numbers are in squares which has a common edge. Then there are two perfect squares on the same line or column. Can you give me an hint? How to start?

from Hot Weekly Questions - Mathematics Stack Exchange

Theorem $\mathbf{A.2.11}$ (Dominated convergence). Let $f_n : X \to \mathbb R$ be a sequence of measurable functions and assume that there exists some integrable function $g : X \to \mathbb R$ such that $|f_n(x)| \leq |g(x)|$ for $\mu$-almost every $x$ in $X$. Assume moreover that the sequence $(f_n)_n$ converges at $\mu$-almost every point to some function $f : X \to \mathbb R$. Then $f$ is integrable and satisfies $$\lim_n \int f_n \, d\mu = \int f \, d\mu.$$

I wanted to know if in the hypothesis $|f_n(x)| \leq|g(x)|$ above, if I already know that each $f_n$ is integrable, besides convergent, the theorem remains valid? Without me having to find this $g$ integrable?

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/regi_zteel
[link] [comments]

from math https://ift.tt/2YASgbg

So I was reading about the ZFC axioms, and apparently some of them are actually "axiom schemas." For example, there is the "axiom schema of specification," which basically says that give a set $A$ and a formula $\phi(x)$, a subset of $A$ exists where all the elements satisfy $\phi(x)$.

This is apparently not one axiom, but a schema of infinitely many axioms, because there is one axiom for every $\phi(x)$. So that must mean that for whatever reason, just letting $\phi(x)$ be an arbitrary formula does not make a valid axiom. So are there rules for what an axiom can say?

So my questions are: Why is this not allowed to be one axiom? What are the rules for what an axiom is allowed to be? And why?

from Hot Weekly Questions - Mathematics Stack Exchange

Find all functions $f:\Bbb{R} \to \Bbb{R}$ such that for all $x,y,z \in \Bbb{R} $ , $f(f(x)+yz)=x+f(y)f(z)$

I was told to do this by proving $f$ is injective and surjective. I have proved it this way : setting $y=z=0 $, and then $f(f(x))=x+f^2(0)$. For any $b \in \Bbb{R}$, $x+f^2(0)=b$ has a solution ,then $f(f(x))=b$ has a solution and it follows that $f$ is surjective. For $f(x)=f(y)$, $f(f(x))=f(f(y))$, so $x+f^2(0)=y+f^2(0)$ , so $x=y$. That's $f$ is injective. But how to find $f$ , I have no idea.

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/matqkks
[link] [comments]

from math https://ift.tt/2UmN37M

March 28th was opening day for this year's Major League Baseball season. It's a good time for some Major League Baseball math! In this activity students think about how major league game lengths have changed over time. Is there a need to pick up the pace of the game?  Have baseball games been getting longer?  If so, why is that?

Students examine two scatter plots and a line graph to decide how the average length of time for an MLB game has changed and by what rate.  As they analyze the causes for that change they observe the average number of pitchers per game, historically, and how the ratio of foul balls to balls in play has changed.

Before handing out the activity consider starting by asking student how long they think a Major League Baseball game takes to play.  Do they think games are taking longer or are they getting shorter and why?

Here is a great video that you and your class can watch right after the first scatter plot. Just be aware, that at 2:04 in the video, David Ortiz is bleeped out saying "bullsh__".    https://youtu.be/RlSEE0a7xY8

And here is a great article - "Foul Balls Are the Pace-of-Play Problem That No One Is Talking About".

The activity: Are-baseball-games-getting-longer.pdf

This activity could be used with any age group from 5th grade up if you don't emphasize a line of best fit.

CCSS: 8.SP.1, 8.SP.2, 8.SP.3, HSS.ID.B.6, HSS.ID.C.7, You could also address with tech: HSS.ID.C.8

For members we have an editable Word docx, our Excel sheets of data, and solutions.

Are-baseball-games-getting-longer.docx     MLB-games-getting-longer.xlsx

Are-baseball-games-getting-longer-solution.pdf

from Yummy Math

Function $f$ is continuous and strictly increasing on $[a,b]$ and $g$ is Riemann integrable such that $g \circ f$ is defined. Is $g \circ f$ Riemann integrable?

I was able to show that if $f$ additionally satisfies:
$x_{1},x_{2} \in [a,b] \Rightarrow f(x_2)-f(x_1) \geq x_{2}-x_{1}$, then $g \circ f$ is Riemann integrable.

from Hot Weekly Questions - Mathematics Stack Exchange

Tamil Nadu State Board 10th Maths Solution

from Math Blog https://ift.tt/2V7OXpU

Reducing Rational Expressions to Lowest Terms

from Math Blog https://ift.tt/2FFBOOp

Finding LCM and HCF of Polynomials and Relationship Worksheet

from Math Blog https://ift.tt/2I2F1du

Arcs and Chords

from Math Blog https://ift.tt/2V3mm4R

How to Find lcm From Two Polynomials and gcd

from Math Blog https://ift.tt/2JS1zQG

This identity was posted a while back but the question had been closed; the question wasn't asked elaborately, though the proof of the identity is a nice application of combinatorics and a good example for future reference.

Also, there was just a flat-out wrong answer which had a couple of upvotes, so I thought to give my own possible proof and sort of 'reopen' the question that was left. Hopefully this time the question will have enough context to stay alive.

As a side note: the given right side does not appear symmetric between $k$ and $n$. However, when $\binom{m}{2}=m(m-1)/2$ is inserted and the products expanded, only symmetric terms remain uncancelled. Rendering the right side as $k^2\binom{n}{2}+n\binom{k}{2}$ would give the same cancellations and the same net value.

Proof

Suppose you have a grid of $n\times k$ dots. Firstly, the amount of ways to connect any two dots is $\binom{nk}{2}$. Now consider the right-hand side. We can split the cases for which the connected dots are on the same column, row, or are in both different columns and rows.

If the two connected dots are in the same column, we can choose two points from any two different rows in $\binom{n}{2}$ ways, and we have $k$ columns for which the two points can be on the same column, which gives a total of $k\binom{n}{2}$ options. The same argument holds for constant rows: $n\binom{k}{2}$.

Now if neither the row nor the column can stay constant, we can pick any point in $nk$ ways, and choose the second point from the remaining $(n-1)(k-1)$ points; one column and one row will be unavailable. This gives us $\frac{nk(n-1)(k-1)}{2}$ options, as we have to rule out the double counting.

We will now show (algebraically) that $n\binom{k}{2}+\frac{nk(n-1)(k-1)}{2}=n^2\binom{k}{2}$. We have that $\binom{k}{2}=\frac{k(k-1)}{2} =\frac{nk(n-1)(k-1)}{2n(n-1)} \iff n(n-1)\binom{k}{2}=\frac{nk(n-1)(k-1)}{2}=n^2\binom{k}{2}-n\binom{k}{2}$ which leads to the equation above.

Combining these cases gives $\binom{nk}{2}=k\binom{n}{2}+n\binom{k}{2}+\frac{nk(n-1)(k-1)}{2} = k\binom{n}{2}+n^2\binom{k}{2}$

If there are any mistakes or improvements on the arguments, please feel free to point them out.

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/baristamathematician
[link] [comments]

from math https://ift.tt/2FMPnwJ

We say that a given theory $T$ admits QE in a language $\mathcal{L}$ if for every $\mathcal{L}$-formula, there is an equivalent quantifier free $\mathcal{L}$-formula. That is for every $\mathcal{L}$-formula $\phi(x)$, where $x$ is a free variable, there is an $\mathcal{L}$-formula $\psi(x)$ so that $T\vDash\forall x\left(\phi(x)\iff\psi(x)\right)$.

The way I interpret this is that for any formula which $T$ implies, there is an equivalent q-free formula which $T$ imples. In other words, all the logical consequences of $T$ are expressible q-free.

My question is then:

Why is this advantageous? What is the benefit of having every logical consequence of a theory being q-free expressible?

Wikipedia says something along the lines that admitting QE makes the decidability problem simpler. But doesn't every theory admit QE in a sufficienctly complex language? Why is it desirable to be decidable with respect to a small (simpler) language?

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/AZiada
[link] [comments]

from math https://ift.tt/2I2k3vi

submitted by /u/philgabrielpeter
[link] [comments]

from math https://ift.tt/2JRzgSz

submitted by /u/Charmxnder
[link] [comments]

from math https://ift.tt/2OzT6jU

If $d$ divides $f(a)=a^4+a^3+2a^2-4a+3$, prove that $d$ is a fourth power modulo $13$.

$f(a)\equiv{(a-3)}^4\pmod 13$. But how can we prove any divisor of $f(a)$ is a fourth power? If we prove that any prime divisor $p$ of $f(a)$ is a fourth power modulo $13$, we would be done as the fourth powers form a group under multiplication modulo $13$.

If we can write $f(a)=P(a)^2-13Q(a)^2$ for some polynomials $P(a)$ and $Q(a)$, $p$ divides $f(a)$ implies $13$ is a square modulo $p$ and quadratic reciprocity will imply $p$ is a square modulo $13$. I could not find suitable $P(a)$ and $Q(a)$ but I think this will help. To prove fourth power, I think quartic reciprocity will help, but I don't know.

Any help will be appreciated.

from Hot Weekly Questions - Mathematics Stack Exchange

$\sin^2(x)$ has period $\pi$ but it seems to me $\sqrt{\sin x}$ is not periodic since inside square root has to be positive and when it is negative, it is not defined.

Does it creates problem for periodicity? Can we say square root of the periodic function need to be periodic? Thanks for your help.

This is graph of the $\sqrt{\sin x}$ above.

from Hot Weekly Questions - Mathematics Stack Exchange

We have 4 dogs and 3 cats in a line but no two cats can be together, in how many ways can they be arranged?

Since there are 5 spaces the cats can be in with the dogs fixed, there are ${5 \choose 3} * 4! * 3! = 1440$ ways and this is the correct answer.

I thought of a different approach. Instead of fixing the dogs' places, I fixed the places of the cats. Now, we have 4 spaces of which the two spaces in the middle must be filled. Therefore, out of the 4 dogs, 2 must fill those, and there are $4 * 3$ ways of doing this (since one dog must be chosen to fill one middle space and the other, to fill the second but now there are only 3 dogs left.)

The other two dogs are free to go to any of the 4 spaces, with $4^2$ possibilities.

The cats can now be arranged in $3!$ ways.

So, our final answer should be $3! * 4^2 * 4 * 3 = 1152$

Where have I gone wrong?

from Hot Weekly Questions - Mathematics Stack Exchange

Evaluate: $\lim_{n \to \infty}\displaystyle\sum_{r=1}^{n} \dfrac{1}{2n + 2r-1}$

To solve this I used the following approach: $S = \lim_{n \to \infty}\displaystyle\sum_{r=1}^{n} \dfrac{\frac 1n}{2 + 2\frac rn-\frac1n}$

$= \lim_{n \to \infty}\displaystyle\sum_{r=1}^{n} \dfrac{\frac 1n}{2 + 2\frac rn-\color{red}{0}} = \dfrac 12\int _0^1 \dfrac{1}{1+x}\mathrm{dx} = \ln(\sqrt 2)$

Though the answer is correct, I am unsure about my second step in which I have replaced $1/n$ by $0$. Is that allowed? I have tried it in some other problems too and it works.

from Hot Weekly Questions - Mathematics Stack Exchange

First-order partial derivatives of functions with two variables. Dear friends, today’s topic is first-order partial derivatives of functions with two variables. In general, we all have studied partial differentiation during high school. So this is more like a re-visit to the good old topic. Have a look!! First-order partial derivatives of functions with two variables Suppose...

The post First-order partial derivatives of functions with two variables appeared first on Engineering math blog.

from Engineering math blog https://ift.tt/2HLzLMh

How to Find GCD and LCM of Two Polynomials

from Math Blog https://ift.tt/2V1Kt4c

Finding gcd of Polynomials by Long Division Worksheet

from Math Blog https://ift.tt/2U3BuTA

Solving Linear Systems in Three Variables Worksheet

from Math Blog https://ift.tt/2Uj5Cth

lcm of Polynomials by Factoring

from Math Blog https://ift.tt/2JRCUMp

GCD of Polynomials Using Division Algorithm

from Math Blog https://ift.tt/2FFSaX3

It is proved that the Poincaré inequality is still true for functions with zero mean boundary traces. Motivated by this, I have the following question:

Let $\Omega$ be an open,bounded and connected subset of $\mathbb R^3$ with a $C^2-$boundary $\partial \Omega \equiv \Gamma$. If $f \in W^{1,2}(\Omega)$ then could we claim that:

${\vert \vert f - \frac{1}{\vert \Gamma \vert } \int_{\Gamma} {\vert f \vert}^{1/2} \vert \vert}_{L^2(\Omega)} \leq C {\vert \vert \nabla f \vert \vert}_{L^2(\Omega)}$

If for any $u\in \{ u\in H^1(\Omega): \frac{1}{\vert \Gamma \vert } \int_{\Gamma} u=0 \}$ we have the estimate: ${\vert \vert u \vert \vert}_{L^2(\Omega)} \leq C {\vert \vert \nabla u \vert\vert}_{L^2(\Omega)}$ then it seems logical to me that the above claim could be true. However I wasn't able to prove it (if indeed can be proved) so any help is much appreciated.

Thanks in advance!

from Hot Weekly Questions - Mathematics Stack Exchange

Word Problems Involving Linear Equations in Three Variables

from Math Blog https://ift.tt/2CKGUZ7

Discuss the Nature of Solutions of Linear Equations in Three Variables

from Math Blog https://ift.tt/2HZx0WQ

submitted by /u/SophoterosAmy
[link] [comments]

from math https://ift.tt/2uAlysQ

submitted by /u/ric05712
[link] [comments]

from math https://ift.tt/2uIzQb3

Show that if $a_n,b_n\in\mathbb{R}$, $(a_n+b_n)b_n\neq0$ and both $\displaystyle\sum_{n=1}^{\infty}\frac{a_n}{b_n}$ and $\displaystyle\sum_{n=1}^{\infty}\left(\frac{a_n}{b_n}\right)^2$ converge, then $\displaystyle\sum_{n=1}^{\infty}\frac{a_n}{a_n+b_n}$ converges.

If $a_n$ is positive, I have been able to solve. How we can solve in general?

from Hot Weekly Questions - Mathematics Stack Exchange

Finding value of $\displaystyle \int^{\infty}_{0}\frac{x\sin x}{(x^2+1)^3}dx$

Let $$ I = \frac{1}{2}\int^{\infty}_{0}\sin x\frac{2x}{(x^2+1)^3}dx$$

Integrating by parts

$$ \Rightarrow I = -\frac{\sin x}{(x^2+1)^2}\bigg|^{\infty}_{0}+\int^{\infty}_{0}\frac{\cos x}{(x^2+1)^2}dx=\int^{\infty}_{0}\frac{\cos x}{(x^2+1)^2}dx$$

How do i solve it?

from Hot Weekly Questions - Mathematics Stack Exchange

I ran into two very similar problems concerning quadratic residues, and I'm having a bit of trouble working through them. These problems are supposed to rely exclusively on the theory of cyclic groups, without use of Legendre symbols. I'm posting both in one question since I roughly managed to solve the first one and it's meant to show my thought process towards solving the second.

Let $p$ be a prime congruent to $1$ modulo $3$. Show that there exists an $a \in \mathbb{Z}$ such that $a^2 + a + 1 \equiv 0 \textrm{ mod } p$ and conclude that $-3$ is a square modulo $p$.

To solve this one, I let $p = 3k + 1$, and take $g \in (\mathbb{Z}_p, \times)$ to be a generator from which follows that $g^{3k} - 1 = (g^k - 1)(g^{2k} + g^k + 1) \equiv 0 \textrm{ mod } p$. Since $g$ is a generator, $g^k \ne 1$. To conclude $-3$ is a square, I (somewhat randomly) noticed that

\begin{align*} (g^k - g^{-k})^2 &= g^{2k} - 2 + g^{-2k} \\ &= g^{2k} + g^k + 1 - 3 \\ &\equiv -3 \textrm{ mod } p \end{align*}

I was wondering, is there any significance to the element $g^k - g^{-k}$ as a root for $-3$? Is there any way to intuitively know immediately that's the square you're looking for? I remember seeing similarly defined elements before, and I pretty much just plugged it in hoping for the best, without really knowing what I was doing. The next problem has me completely stumped.

Let $p$ be a prime congruent to $1$ modulo $5$. Show that there exists an $a \in \mathbb{Z}$ such that $(a + a⁴)² + (a + a⁴) - 1 \equiv 0 \textrm{ mod } p$ and conclude that $5$ is a square modulo $p$.

I sort of have this sense that I'm gonna need an element of order $10$, i.e. $g^{\frac{5k}{2}}$ where $g$ is yet again a generator, however I can't seem to get anywhere with this. If I let $x = a + a^4$, then I can tell I'm basically looking for an element $x$ which has the next element $x + 1$ as its inverse, but that doesn't really help me forward.

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/AutoModerator
[link] [comments]

from math https://ift.tt/2uxVlLh

submitted by /u/accessdeniedr99
[link] [comments]

from math https://ift.tt/2THYEcX

from Maths & Physics News

     Celebration is everywhere (including here in The New Yorker ) -- mathematician Karen Uhlenbeck has recently won the Abel prize for her revolutionary work: " . . . pioneering achievements in geometric partial differential equations, gauge theory and integrable systems, and for the fundamental impact of her work on analysis, geometry, and mathematical physics."
     Here (pulled from The New Yorker article also cited above) are some of Uhlenbeck's poetic words about women in mathematics:

       It's really hard for me to describe
          to people who are not somewhat near me in age
       what it was like for women then ... and it was only
          because of the women's movement and books like  
Read more »

from Intersections -- Poetry with Mathematics

I am facing difficulty in solving the 18th Question from the above passage.

Attempt:

I have attempted it using principal of inclusion and exclusion.

Let n be the required number of ways.

$n = \text{Total number of ways - Number of ways in which Os and Is are together}$

$\implies n = \dfrac{12!}{3!2!2!} - \dfrac{11!}{2!3!} - \dfrac{10!}{2!2!}+ \dfrac{9!2!}{2!} = 399 \times 8!$

This gives $N = 399$ which is wrong. Can you please tell me why am I getting the wrong answer?

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/Fly_GP
[link] [comments]

from math https://ift.tt/2uwar4d

I want to transform the following $$\prod_{k=0}^{n} (1+x^{2^{k}})$$ to the canonical form $\sum_{k=0}^{n} c_{k}x^{k}$

This is what I got so far \begin{align*} \prod_{k=0}^{n} (1+x^{2^{k}})= \dfrac{x^{2^{n}}-1}{x-1} (x^{2^{n}}+1) \\ \end{align*} but I don't know how to continue, can someone help me with this?

from Hot Weekly Questions - Mathematics Stack Exchange

Has $$2^{n-1}\equiv 2^{41}+1\mod n$$ a solution with a positive integer $\ n>1\ $ ?

Motivation : The equation $$2^{n-1}\equiv k\mod n$$ has always a solution, if $\ k-1\ $ has an odd prime factor (this odd prime factor is then a solution) and for $\ k=2^m+1\ $ , I know a solution for $$m=1,2,3,\cdots,40$$ Hence, this is the smallest number for which I know no solution. Upto $\ n=10^9\ $, there is no solution.

from Hot Weekly Questions - Mathematics Stack Exchange

Assume $f:\mathbb{R}_{>0}\to\mathbb{R}_{>0}$ is a continuous function such that $xf(f(f(x)))=1$ for all $x>0$.

I found that $f(x)=1/x$ is a solution. Could we find another such function? And why?

from Hot Weekly Questions - Mathematics Stack Exchange

Hello fellow math educators! We hope you will be joining us in San Diego, CA for the NCTM conference next week. If you’re going, make sure these thought-provoking sessions with our authors and professional learning experts make it onto your schedule. Visit our booth #1225 for fun activities and delicious treats, and take a moment to…

from Math Solutions https://ift.tt/2FAxj7H

Source: https://scontent-dfw5-1.xx.fbcdn.net/v/t1.0-9/44665774_2388027201214092_5864075778743861248_n.jpg?_nc_cat=100&_nc_ht=scontent-dfw5-1.xx&oh=ee5e18af1ccda3f69c03ed9bdc35a280&oe=5CA63AEC

from Mean Green Math

Solving Linear Equations in Three Variables Example Problems

from Math Blog https://ift.tt/2WwBbgF

Solving System of Linear Equations in Three Variables

from Math Blog https://ift.tt/2OxsOP9

We tell the following to our Calc III students (usually for $\mathbf{R}^2$, and never so formally):

Let $A$ be an open subset of $\mathbf{R}^n$, $a\in A$, $f$ a real-valued function on $A$ and $\Gamma = \{\gamma \in A^{[0,1]} : \gamma(0) = a$ and $\gamma$ is continuous at $0\}$. If 𝑓∘𝛾 is continuous at $0$ for all $\gamma \in \Gamma$, then $f$ is continuous at $a$.

We may generalize this: $A$ can be a first countable locally path-connected space, and the codomain may be any topological space. See Continuity on paths implies continuity on space? .

Here is my question:

Does one need the Axiom of Choice (or at least countable choice) to prove this result?

For example, the essentials of my proof of the (restricted) result is: If $f$ is not continuous at $a$, then there is a neighborhood $V$ of $f(a)$ such that $f^{-1}(V)$ is not a neighborhood of $a$. For each positive integer $n$, choose a point in $ B(a,1/n)\backslash f^{-1}(V)$ and connect the points with a piecewise linear path. Thus, we are choosing countably many points.

The proof at the link above also uses countable choice.

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/gordon2222
[link] [comments]

from math https://ift.tt/2JXKSDM

Sum of Special Series Worksheet for Grade 10

from Math Blog https://ift.tt/2JPN2oT

Sum of Special Series Examples

from Math Blog https://ift.tt/2TGN9T5

submitted by /u/flexibeast
[link] [comments]

from math https://ift.tt/2WxT7HJ

How to Find Sum of Special Series

from Math Blog https://ift.tt/2FI8GqW

I am totally new to differential geometry and am having trouble understanding a very basic idea. In what follows, I apologize for being gratuitously pedantic, but I want to be sure I clearly understand what's going on.

If $M$ is a set and $T$ is a topology on $M$ such that $(M,T)$ is Hausdorff and second countable, then $M$ is a topological manifold if for all $p\in M$ there exists an ordered pair $(U,x)$ such that $U \subset M$ is $T$-open and $x:U\rightarrow \mathbb{R}^d$ is a homeomorphism whose image is an open subset of $\mathbb{R}^d$ in the standard topology.

Ordered pairs $(U,x)$ that satisfy the conditions in the above paragraph are called charts on the manifold. An atlas for $M$ is a collection of charts on $M$, $A = \{(U_a,x_a)\colon a \in I\}$, such that $\cup_{\alpha\in I}U_a = M$.

Question 1: Does every manifold have at least one atlas?

My answer: I believe so, since by the definition of a manifold there exists at least one chart for each point, and the collection of either all or at least one of the charts at each point can be taken as an atlas. Perhaps however there is some technical problem in set theory with this construction.

Question 2: Does an atlas uniquely define a manifold? That is, if $A$ and $A'$ are atlases and $A \neq A'$, is it necessary true that the manifolds with $(X,T)$ as their underlying space but with atlases $A$ and $A'$ respectively are different? (In the naive sense--not considering the possibility that they are diffeomorphic)

I believe the core concept I'm struggling with here is what the naive notion of equivalence is for manifolds. (For example, for topological spaces "naive equivalence" means that the two underlying sets are equal and the two topologies have exactly the same open sets, rather than the existence of a homeomorphism, which is a more sophisticated notion of equivalence.)

If instead we define a topological manifold as an ordered triple $(M,T,A)$, where $A$ is an atlas, my confusion vanishes. But then naive equivalence requires exactly the same charts in the atlas, which might be too much to reasonably say that two manifolds are the same. I've also not seen this definition in any of the references I'm using. This brings up the following question.

Question 3: Is it possible to define a manifold as an ordered triple, as in the paragraph above?

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/sakshays
[link] [comments]

from math https://ift.tt/2HXH3vy

submitted by /u/Melboul
[link] [comments]

from math https://ift.tt/2JOarHj

Finding Sum of Geometric Series Worksheet

from Math Blog https://ift.tt/2OwBLbH

Difficult Problems on Geometric Series

from Math Blog https://ift.tt/2YvRMD8

Are you ready for NCSM 2019 in San Diego, CA? We can’t wait to see you in our Booth 113 for a Math Workshop Essentials: Developing Number Sense signing with authors Rusty Bresser & Caren Holtzman. We have a full lineup of professional learning sessions for you to check out as well. Hope to see you there!   …

from Math Solutions https://ift.tt/2CIrepg

submitted by /u/pinebug
[link] [comments]

from math https://ift.tt/2uGPT9j

In English, we read and write from left to right, but for some reason we apply functions in the opposite order. Consider the following procedure:

Take an element $x$, apply a function $f$ to it and then apply a function $g$ to the result.

The formula we would write for this is $g(f(x))$, which is in some sense the wrong way around, it would be more consistent to write $((x)f)g$. (I have to admit that I had some trouble formatting that, but that's presumably because I'm used to do it the other way around.) This would not only be consistent with the way we write, but also with the notation using arrows, i.e. \begin{equation} X \overset{f}{\rightarrow} Y \overset{g}{\rightarrow} Z \end{equation}

What is the reason for this apparent inconsistency?

I could imagine the reason to be either historical or logical, or both, and I would be interested in either explanation.

---

I wasn't sure how to tag this, so feel fry to add any appropriate tags.

from Hot Weekly Questions - Mathematics Stack Exchange

Consider the set $A=\{\sqrt{2017+n^2}:n\in N\}$. How many numbers in the set A are rational?

My attempt:

The square-root of a non-negative integer can either be a rational number or an irrational number. When it is a rational number it has to be an integer. It cannot be anything else (I do not exactly know why but my mind says so).

So by this logic, if $\sqrt{2017+n^2},n\in N$ is a rational number , it has to be a positive integer. Therefore, $2017+n^2=k^2$, for some $k\in N$. Therefore, $2017=(k+n)(k-n)$. Since $k,n\in N$, and $2017$ is a prime number, $(k+n)=2017,(k-n)=1$. This implies, $k=1009, n=1008$. Since we got one value of $n$, there is only one number is $A$ which is rational.

Is my reasoning and my answer right? If not then what is the correct reasoning and answer and if yes then what is the justification of the line in bold?

from Hot Weekly Questions - Mathematics Stack Exchange

Two of math education’s most exciting events are taking place next week in San Diego:

• National Council of Supervisors of Mathematics (NCSM) Annual Conference, April 1-3

• National Council of Teachers of Mathematics (NCTM) Annual Conference, April 3-6

The events give educators an opportunity to come together and share their perspectives about issues, challenges, and best practices regarding mathematics education and leadership. MIND Research Institute, creators of ST Math, will be on hand in the exhibit hall and throughout the week to share our views on the future of math education.

Nigel Nisbet, MIND’s Vice President of Content Creation, will be at NCSM for two exciting presentations:

 

Paradigm Shift: Changing the Culture of Mathematics and Learning in Schools (Sponsor Showcase)

Date and time: April 2, 11:15 a.m. to 12:15 p.m.

Location: Torrey Pines 3

Description: Society has a math problem, and it's not one that can be solved by traditional methods. To improve outcomes at a significant scale in our education system, we must become adept at changing the culture of learning. In this presentation, Nigel will demonstrate how neuroscience, motivational research, and innovative technology can transform mathematics and learning for students, teachers, and parents.

 

The Neuroscience of Deeper Learning (Luncheon)

Date and time: April 3, 12:00 to 12:30 p.m.

Location: Grand Ballroom

Description: Advances in brain research have moved educators closer than ever before to teaching math the way the brain actually learns. Journey with Nigel to uncover a neuroscience-based, unifying theory that promotes deeper engagement, deeper thought and deeper learning. He'll share insights on how our brains learn and how to create active classroom environments that foster student success in the 21st century.

For a taste of what you can expect at the conference next week, take a peek at Nigel’s TEDxOrangeCoast presentation: “The Geometry of Chocolate.” 

During NCTM, please join Erich Zeller, Instructional Designer at MIND, for his session:

 

Math in the 21st Century Classroom (Exhibitor Workshop)

Date and time: April 4, 11:00 a.m. to 12:00 p.m.

Location: Room 33 C

Description: In order for students to truly gain deep conceptual understanding, they must be able put ideas into words, as well as explain and justify their reasoning. Erich will share ways that educators and educational environments can foster meaningful discourse, problem solving, and communication in the classroom.

 

And stop by our booth to see ST Math in action and meet the team!

• Booth #212 at NCSM

• Booth #604 at NCTM

We hope to see you there!

If you can’t make it to the conference, you can still keep up with the fun on Twitter @MIND_Research and @stmath. Follow all the action at #NCTMSD2019 and #NCSM19.

from MIND Research Institute Blog https://ift.tt/2FIPlG5

Let $f:[a,b]\to\mathbb{R}$ be differentiable on $[a,b]$, with $\lim\limits_{x\to a}f(x)=\lim\limits_{x\to a}f^\prime(x)=0$, and $f^\prime(x)\ne 0$ in a neighborhood of $a$. Is it necessarily true that

$$\lim_{x\to a}\frac{f(x)}{f^\prime(x)}=0$$

It doesn't seem necessarily clear to me that this limit should always exist, and that there's not some pathological function for which the limit is nonzero.

from Hot Weekly Questions - Mathematics Stack Exchange

submitted by /u/Candpolit
[link] [comments]

from math https://ift.tt/2Wva5qw

Check out this Problem of the Week.
Be sure to let us know how you solved it in the comments below or on social media!
Solution below.



from The Center of Math Blog https://ift.tt/2V2kcmb

How to Find the Sum of Special Type of Geometric Series

from Math Blog https://ift.tt/2YrAHKN

The representations of a finite group can be understood by their irreducible characters. A class function is a function from the group to the complex numbers that is constant on the conjugacy classes.

I know that any linear combination of the irreducible characters is the character of some representation. I also know that not all class functions are characters of a representation.

Let's say that I don't know all the irreducible characters of a group, but I come across a class function whose inner product with itself is 1. My question is: How do I know whether this function is actually the character of an irreducible representation?

More generally: How do I know whether a given class function is the character of some representation of a group without knowing all the irreducible representations?

EDIT: I see this question with answers: Class function as a character. This almost answers my question. To clarify what I am specifically interested in knowing, if I have found some irreducible representations of a group $G$. Say I have $\chi_1, \dots, \chi_m$. I know I haven't found all of them because I know the number of conjugacy classes. Then, say, I some other non-irreducible character $\chi$ and I know, say, that this is the character of some representation. Then I subtract a linear combination of $\chi_1, \dots, \chi_m$, and define the class function $\psi = \chi - (a_1\chi_1 + \dots + a_m\chi_m)$. How do I know whether this $\psi$ is the character of some representation?

from Hot Weekly Questions - Mathematics Stack Exchange

Is there a general method for testing numbers to see if they are perfect $n$th powers?

For example, suppose that I did not know that $121$ was a perfect square. A naive test in a code might be to see if $$\lfloor\sqrt{121}\rfloor=\sqrt{121}$$

But I imagine there are much more efficient ways of doing this (if I'm working with numbers with many digits).

from Hot Weekly Questions - Mathematics Stack Exchange

How to Find the Sum of n Terms in a Geometric Series

from Math Blog https://ift.tt/2uyMLMA

$A'$ is a moving point of side $BC$ of $\triangle ABC$. The perpendicular bisector of $A'B$ and $A'C$ cuts side $AB$ and $AC$ respectively at $B'$ and $C'$. Line $d$ passes through $A'$ and is perpendicular to $B'C'$. Prove that $d$ passes through a fixed point.

I have predicted that $d$ would pass through point $A''$ in which $AA'' \perp BC$ and $A''$ lies on the circumcircle of $\triangle ABC$. But I haven't found out a way to prove that yet.

from Hot Weekly Questions - Mathematics Stack Exchange

$2^\sqrt2$ vs $e$, which is greater?

$(2^\sqrt2)^\sqrt2 = 4\quad $ & $\quad e^\sqrt2$ = ?

$\log(2^\sqrt2) = \sqrt2\log(2)\quad$ & $\quad \log(e) = 1$

I tried but can't induce comparable form.

Is anybody know how to prove it?

from Hot Weekly Questions - Mathematics Stack Exchange

What are the issues surrounding the use of realistic contexts in the mathematics classroom?

from Cambridge Mathematics News https://ift.tt/2I1PMwV

The paper “The boundary layer flow induced above the torsional motion of a disk” co-authored by Matt Turner and Patrick Weidman (University of Colorado) has been accepted for publication in Physics of Fluids. The paper presents an exact solution to the axisymmetric boundary-layer equations above a twisting elastic disk. The results are presented in terms of the twisting parameter m>1 and it is predicted that the flow becomes more stable as m increases due to the boundary layer thinning in this limit. The author final copy of the paper can be found here.

from Surrey Mathematics Research Blog https://ift.tt/2YwVlsT

28 Mar 2019

In this Newsletter:

1. New on IntMath: Implicit graph plotter
2. Resources: Unofficial Desmos, Observable
3. Math in the news: Navier-Stokes
4. Math movies: Math anxiety, Wind
5. Cattle functions
6. Math puzzle: Repeated digits
7. Final thought: Stats, effort and money

1. New on IntMath: Implicit graph plotter

The majority of the graphs we come across are of explicit functions. These are ones where there is a "y" on the left of the equation, and all the x-terms are on the right. Examples:

y = x²

y = sin(x)

y = 1/x

Such functions have one y-value for each x-value we substitute in. As such, they are relatively easy to graph using computers, because we can choose a set of x-values, substitute them in, and join the resulting (x, y) pairs, similar to the way we sketch graphs on paper.

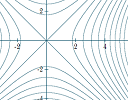
On the other hand, implicit functions appear with x- and y-terms mixed together, and often it's impossible to separate them such that we are left with a simple "y" on the left. Here are some implicit functions:

sin(x² + y³) = √(x + y + xy)

x² + y² = 25 log(x + y)

These implicit functions are much harder to graph using a computer, since we can have a lot more than one value for y for each value of x, so finding all the correct ordered pairs and then joining them can be quite a programming challenge.

I recently added an implicit graph plotter which uses a technique called Interval Arithmetic, that ensures each pixel in a vertical interval is catered for using variable-width x-intervals, and does quite a good job of addressing the many issues involved in graphing implicit functions.

Here's some background and examples: Implicit plots in ASVG via Interval Arithmetic, D3 and Function Plot

You can see it in action on this page:

Differentiation of Implicit Functions (see the main page, and then the answers for Example 2 and 3.)

2. Resources -

(a) Unofficial Desmos

Desmos Graph Calculator is a great tool for exploring mathematics, and for setting up interactive applets for others to use.

Sometimes the documentation for Desmos is not as extensive as you may like.

DesmosGraph – unofficial aims to fill that gap by providing tips and tricks for how to get the most out of Desmos.

(b) Observable

Observable is a "magic notebook for visualization, exploring data, interactive essays and live coding".

The concept is similar to Wolfram's Notebooks (and SageMath), but Observable is free and open source (as is Sage).

Observable is a neat Web-based math coding solution that can produce a wide variety of data visualizations. Go to: Observable

See visualization examples.

Don't miss this demo and these Tutorials.

Observable has a lot of potential for class projects, and for writing up science lab results.

Observable is by Mike Bostock, American computer scientist and data-visualisation specialist and one of the creators of D3.js, a brilliant javascript data visualization library.

3. Math in the news: Navier-Stokes equations under review

The Navier-Stokes equations describe what's going on when fluids move and interact. They are extensively used to model the weather, ocean currents, air moving over aircraft wings and even for simulating effects in computer games.

The equations have been in use since their development in the 19th century, generally with great success. However, there's a problem.

Do they work?

Most observations confirm the Navier–Stokes equations do "work", but mathematically, there are still several doubts about them, precisely because they follow from observation, rather than being the result of mathematical rigor. It's not even known whether the solutions always exist in 3 dimensions, nor whether they are continuous.

There's a $1 million reward for anyone who can mathematically prove they work, or provide an example where they don't.

For an interesting discussion on the issue from Quanta Magazine, see: Mathematicians Find Wrinkle in Famed Fluid Equations

4. Math Movies

(a) Why do people get so anxious about math?

Some studies have shown up to 20% of people suffer from math anxiety. We're not talking about the usual butterflies we all get when facing a test.

Math anxiety is a serious, debilitating condition that has enormous implications for people. See: Why do people get so anxious about math?

(b) The Boy Who Harnessed the Wind: William Kamkwamba

Willaim Kamkwamba grew up in the grinding poverty, drought and hunger of Malawi, in southeast Africa.

Thrown out of school because his father couldn't pay the fees, 13 year-old William sets about inventing a wind turbine to power electrical devices, including a water pump.

Through his own grit, inventiveness and willingness to find things out by himself, he has built other wind turbines and a solar power unit.

In 2014 he received a Bachelor of Arts degree in Environmental Studies from Dartmouth College in the UK.

Here's his TED talk: How I harnessed the wind

The BBC recently made an inspiring movie about the story, now showing on Netflix. Here's the trailer: The Boy Who Harnessed the Wind.

5. Cattle functions

I hope these trigger memories next time you need to sketch (or make use of) a polynomial or cosine function.

[Image source]

6. Math puzzles

The puzzle in the last IntMath Newsletter asked about integers with remainders when divided by certain numbers. Correct answers with sufficient reasons were submitted by Chris, Rick, Russell, Thomas, Vijay and Ben.

Historical note: This remainder problem was first stated in the 3rd century Chinese mathematical treatise Sun Zi Suanjing.

A generalization of the problem is known as the Chinese Remainder Theorem. A special case of the theorem asks for the smallest integer giving 1 as a remainder when divided by each of 4, 5 and 6. The LCM of 4, 5 and 6 is 60, so the required number is 61. (All numbers meeting this condition are in the form 60n + 1).

The Chinese Remainder Theorem came to the West via the 11th century Arab scholar Ibn Tahir and then by Leonardo Fibonacci in his early 13th century treatise Liber Abaci.

New math puzzle: repeated digits

A 4-digit number pqrs (where p ≠ 0 and the digits are not necessarily unique) is multiplied by 1999, resulting in a number which ends with 4 repeated digits. Find all possible numbers pqrs.

You can leave your response here.

7. Final thought - statistics, effort and money

The news is full of statistics. Usually it's a simple count of the number who died in an accident, or who were affected by some natural disaster.

I'm always impressed how air crash investigators rush to the scene of an aircraft accident, and how huge resources are invested in the investigation and follow up actions. The aircraft industry is important to all countries, and it's in everyones' interest to ensure safe operation of aircraft. I get that.

However, what of the other deaths - the ones we don't hear about every day on the news. How about:

• Worldwide, tobacco kills more than 7 million people each year. More than 6 million of those deaths are the result of direct tobacco use while around 890 000 are the result of non-smokers being exposed to second-hand smoke. [Source: WHO]
• Worldwide, 3 million deaths every year result from harmful use of alcohol [Source: WHO]

The efforts put into reducing these 10 million deaths (around 10,000 times the number who perish in air accidents annually) is nothing like the relative effort put into solving (for example) the Boeing 737 Max8 issue. Sadly, as is the case for many things, it comes down to the profit involved.

Until next time, enjoy whatever you learn.

from SquareCirclez https://ift.tt/2CJawFZ
via IFTTT

Geometric Sequence Worksheet for Grade 10

from Math Blog https://ift.tt/2TETJcT

Word Problems in Geometric Sequence

from Math Blog https://ift.tt/2JZDBTZ

Intuition says the vector space of real sequences $R^N$ ($N$ the natural numbers, pointwise addition of real coordinates) is not normable. I have found this surprisingly hard to prove.

I am aware that $R^N$ in the product (Tychonoff) topology is not normable (but metrizable i.e. is a Frechet space). This is proven for instance in Aliprantis–Border: Infinite Dimensional Analysis (2006, p. 207), a reference which I found on Is a metrizable topological vector space normable? in the answer by @triple_sec which I understand and appreciate.

However, this leaves open whether a TVS topology on $R^N$ stronger than the product topology could be normable. Here are my attempts to prove there is no norm defined everywhere on $R^N$ making it a TVS with topology at least as strong as the product topology i.e. with continuous coordinate functions.

I aim for an indirect proof: assume there is a norm on $R^N$ and try to get a contradiction.

a) Banach space arguments: complete the normed space and apply Banach space technology like Uniform Boundedness, Open Mapping etc.. I could not find any proof along this line. (In particular, I found it hard to control whether the completion of $R^N$ in the assumed norm would not make the original normed space "bigger" i.e. adding ideal points outside of $R^N$). But since experts in the field might well know a good argument, I mention the attempt.

b) Geometric argument - construct a vector with an infinite norm: begin with the "unit" vectors $e_n = (0,..., 1, 0, ...) \in R^N, n\in N$ with a $1$ in the n-th coordinate and $0$ elsewhere. Rescale every $e_n$ to have the norm $||e_n||=1$ which is just a coordinate change. Now try $v=(1, 2, 3, ...) \in R^N$ to obtain a vector $v$ with norm $||v||\geq n$ $\forall n$ - are we done? I think no, because the norm $||v||$ of a vector $v=(p_1, p_2, p_3, ...)$ with non-negative real coordinates $p_i, i\in N$ is in general (unlike in the standard sequence spaces $l^p$) not a non-decreasing function of its real coordinates. So I wonder how to argue strictly that $||v||\geq n$ $\forall n$. I tried flipping the signs of each coordinate i.e. consider $v=(\pm 1, \pm 2, \pm 3, ...)$ and use the fact that for arbitrary $v, x$ in a normed space one of $||v+x||$ and $||v-x||$ must be not smaller than $||v||$ and $||x||$ (triangle inequality). But to generate an optimal $v$ with maximal (infinite) norm $||v||$ I face (countably) infinitely many flips depending all on each other. I have no idea how to control (countably) infinitely many sign flips to make such an argument rigorous, but perhaps somebody else has.

The idea seems to be included in the answer of @David C. Ullrich to Can the real vector space of all real sequences be normed so that it is complete ? . There is also an answer and a comment by @paul garrett which indicates what I am trying to prove here is an obvious matter of fact to the experts. However LF-spaces are currently too advanced for me, so I tried the same geometric argument one more time, but now "weakly":

c) Geometric argument - construct a "weakly unbounded" vector: we start again with unit vectors $e_n, n\in N$ like in b), and take the dual sequence of linear functionals $p_n\in (R^N)^*, \forall n \in N$ projecting an arbitrary vector $v$ to its $n$th coordinate. These coordinate functionals are continuous by assumption, and since $p_n(e_n)=1$, the functional norm $||p_n||$ is $\geq 1$. Now we consider the vector $v=(1||p_1||, 2||p_2||, 3||p_3||, ..., n||p_n||, ...)$ and estimate $||v||$ from below: $||v|| \geq |p_n(v)|/||p_n|| =n$ $\forall n \in N$.

My question: is the geometric argument in (c) good enough for a reasonable proof?

Remark upon my original motivation: reading that a product of barreled spaces is barrelled - "Un produit d'espaces tonnelés est tonnelé." on the french https://fr.wikipedia.org/wiki/Espace_tonnel%C3%A9 (the fact is not yet on the english page https://en.wikipedia.org/wiki/Barrelled_space) I tried to prove this. I looked at $R^N$ as one of the simplest non-trivial examples of a product of barrelled spaces: are in there any other barrels except closures of finite products of convex open balls=intervals (all remaining factors equal $R$)? Answer: there are not.

For the ingeneous Bourbaki this was so obvious to write in http://www.numdam.org/article/AIF_1950__2__5_0.pdf on page 6 mere one sentence:

"On montre sans peine que ... tout produit d'espaces tonnelés est tonnelé."

from Hot Weekly Questions - Mathematics Stack Exchange