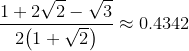What was Galileo’s great innovation in science? To give practical experience more authority than philosophical systems? To insist on mechanical as opposed to teleological or supernatural explanations of natural phenomena? To take mathematical physics as our best window into the fundamental nature of reality as opposed to just a computational tool for a small set of technical problems? No, none of the above. All of these things had been old hat for thousands of years.
Transcript
To say that Galileo is “the father of modern science” is to say that he made some kind of unique contribution, something unprecedented, that was the starting point of science as we know it. So what would that have been? What was that uniquely Galilean ingredient, that made science appear out of thin air for the first time in human history? We spoke about this before. People have tried to pinpoint it in various ways. I refuted the main attempts: mathematisation of nature, empiricism, experimental method. Basically, those things were all commonplace already in Greek times. That’s what I argued last time. But the list goes on. There are other things that Galileo was allegedly “the first” to do. Let’s have a look at those.
Here’s one: Galileo’s greatness consists in bringing together abstract mathematics and science with concrete technology and practical know-how of craftsmen and workers in mechanical fields. Here are some quotes from various historians expressing this idea:
“Real science is born when, with the progress of technology, the experimental method of the craftsmen overcomes the prejudice against manual work and is adopted by rationally trained university-scholars. This is accomplished with Galileo.”
“[Galileo was able] to bring together two once separate worlds that from his time on were destined to remain forever closely linked—the world of scientific research and that of technology.”
“Galileo may fruitfully be seen as the culmination point of a tradition in Archimedean thought which, by itself, had run into a dead end. What enabled Galileo to overcome its limitations seems easily explicable upon considering Galileo’s background in the arts and crafts.”
“The separation between theory and practice, imposed by university professors of natural philosophy, was repeatedly exposed as untenable. Of course the greatest figure in this movement is Galileo.”
So those are four historians all saying basically the same thing.
And Galileo himself eagerly cultivated this image. The very first words of his big book on mechanics are devoted to extolling the importance for science of observing “every sort of instrument and machine” in action at the “famous arsenal” of Venice. He praises the experiential knowledge of the “truly expert” workmen there. Galileo loves these workers and craftsmen in inverse proportion to how much he hates philosophers.
It is true that universities were filled with many blockheads who foolishly insisted on keeping intellectual work aloof from such connections to the real world. For example, when Wallis went to Oxford in 1632 there was no one at the university who could teach him mathematics. As he says in his autobiography: “For Mathematicks, (at that time, with us) were scarce looked upon as Accademical studies, but rather Mechanical; as the business of Traders, Merchants, Seamen, Carpenters, Surveyors of Lands, or the like.”
That was indeed a lamentable state of affairs. But it would be mistake to infer from this that Galileo’s step was an innovation. The stupidity of the university professors was the doing of one particular clique of mathematically ignorant people. Their attitude is not natural or representative of the state of human knowledge. Galileo is not a brilliant maverick thinking outside the box. Rather, he is merely doing what had, among mathematically competent people, been recognised as the natural and obviously right way to do science for thousands of years. Galileo is not taking a qualitative leap beyond limitations that had crippled all previous thinkers. Rather, he is merely reversing the obvious cardinal error of one particularly dumb philosophical movement that had happened to gain too much influence at the time, because people were too ignorant to recognise the evident superiority of more mathematical and scientific schools of thought that had already proven their worth in a large body of ancient works available to anyone who cared to read.
In order to defend the misconceived idea of Galileo the trailblazing innovator one must ignore the large body of obvious precedent for his view in antiquity, and project the foolish nonsense of medieval universities onto the Greeks. Indeed, historians have concocted a false narrative to this effect. Here are some typical quotes:
“Greek technology and science were rigidly separated.”
“The Greek hand worker was considered inferior to the brain worker or contemplative thinker. So, despite the fact that the philosophers derived some of their conclusions as to how nature behaved from the work of the craftsmen, they rarely had experience of that work. What is more, they were seldom inclined to improve it, and so were powerless to pry apart its potential treasure of knowledge that was to lead to the scientific revolution in the Renaissance.”
Others have argued that “the fundamental brake upon the further progress of science in antiquity was slave labour [which precluded any] meaningful combination of theory and practice.”
More specialised scholarship knows better. The recent Oxford Handbook of Engineering and Technology in the Classical World is perfectly clear on the matter:
“Many twentieth-century scholars hit upon [snobbish contempt for manual labour] as an ‘explanation’ for a perceived blockage of technological innovation in the Greco-Roman world. The presence of slave labor was felt to be a related, concomitant factor. [But] this now discredited interpretation [should be rejected and we should] put an end to the myth of a ‘technological blockage’ in the classical cultures.”
This is the view of experts on the matter, while the false narrative is promulgated by scholars who focus on Galileo, take it for granted that he is “the Father of Modern Science,” and postulate such nonsense about the Greeks because that’s the only way to craft a narrative that fits with this false assumption.
Promulgators of the nonsense about practice-adverse Greeks have evidently not bothered to read mathematical authors. Pappus, for example, explains clearly that mathematicians enthusiastically embrace practical and manual skills:
“The science of mechanics has many important uses in practical life, and is zealously studied by mathematicians. Mechanics can be divided into a theoretical and a manual part; the theoretical part is composed of geometry, arithmetic, astronomy and physics, the manual of working in metals, architecture, carpentering and painting and anything involving skill with the hands.”
Pappus praises the interaction of geometry with practical fields or “arts” as beneficial to both:
“Geometry is in no way injured, but is capable of giving content to many arts by being associated with them, and, so far from being injured, it is obvious, while itself advancing those arts, appropriately honoured and adorned by them.”
These were no empty words. The Greeks had an extensive tradition of studying “machines,” meaning devices based on components such as the lever, pulley, wheel and axle, winch, wedge, screw, gear wheel, and so on. The primary purpose of these machines was that of “multiplying an effort to exert greater force than can human or animal muscle power alone.” Such machines were “used in construction, water-lifting, mining, the processing of agricultural produce, and warfare.”
The Greeks also undertook advanced engineering projects, such as digging a tunnel of more than a kilometer through a mountain, the planning of which involved quite sophisticated geometry to enable the tunnel to be dug from both ends, with the diggers meeting in the middle. In short, “while it is crucial to distinguish between theoretical mechanics and practitioners’ knowledge, there is substantial evidence of a two-way interaction between them in Antiquity.”
Mathematicians were very much involved with such things. There are many testimonies attributing to Archimedes various accomplishments in engineering, such as moving a ship singlehandedly by means of pulleys, destroying enemy ships using machines, building a screw for lifting water, and so on. Apollonius wrote a very advanced and thorough treatise on conic sections, which is studiously abstract and undoubtedly “art for the sake of art” pure mathematics if there ever was such a thing. Yet the same Apollonius “besides writing on conic sections produced a now lost work on a flute-player driven by compressed air released by valves controlled by the operation of a water wheel.” The title page of the Arabic manuscript that has preserved this work for us reads: “by Apollonius, the carpenter, the geometer.” The cliche of Greek geometry as nothing but abstruse abstractions divorced from reality is a modern fiction. The sources tell a different story. It is not for nothing that one of the most refined mathematicians of antiquity went by the moniker “the carpenter.”
Unfortunately, as Russo has observed in his excellent book, “Renaissance intellectuals were not in a position to understand Hellenistic scientific theories, but, like bright children whose lively curiosity is set astir by a first visit to the library, they found in the manuscripts many captivating topics, especially those that came with illustrations. The most famous intellectual attracted by all these ‘novelties’ was Leonardo da Vinci. Leonardo’s ‘futuristic’ technical drawings … was not a science-fiction voyage into the future so much as a plunge into a distant past. Leonardo’s drawings often show objects that could not have been built in his time because the relevant technology did not exist. This is not due to a special genius for divining the future, but to the mundane fact that behind those drawings there were older drawings from a time when technology was far more advanced.”
The false narrative of the mechanically ignorant, anti-practical Greeks has obscured this fact, and led to an exaggerated evaluation of Renaissance technology, such as instruments for navigation, surveying, drawing, timekeeping, and so on. Here for example is the view of Jim Bennett, a former Director of the Museum of the History of Science in Oxford:
“Renaissance developments in practical mathematics predated the intellectual shifts in natural philosophy. Historians of the early modern reform of natural philosophy have failed to appreciate the significance of the prior success of the practical mathematical programme, [which] must figure in an explanation of why the new dogma of the seventeenth century embraced mathematics, mechanism, experiment and instrumentation.”
Bennett proves at length that the practical mathematical tradition had much to commend it, which I do not dispute. But then he casually asserts with hardly any justification that there was nothing comparable in Greek times. This is typical of much scholarship of this period. The deeply entrenched standard view of the Galilean revolution is basically taken for granted and subsequent work is presented as emendations to it. For instance, if you want to prove the importance of a Renaissance pre-revolution in practical mathematics, you need to prove two things: first that it was relevant to the scientific revolution, and second that it was not present long before. It is a typical pattern to see historians put all their efforts toward proving the first point, and glossing over the second point in sentence or two. They can get away with this since the alleged shortcomings of the Greeks is supposedly common knowledge, while the first point is the one that departs from the standard narrative. Hence, if the standard narrative is misconceived in the first place, so is all this more specialised research, which, although it ostensibly departs from the standard view, actually retains its most fundamental errors in the very framing of its argument.
It is right to emphasise that the practical mathematical tradition stood for a much more fruitful and progressive approach to nature than that dominant among the philosophy professors of the time. But it is a mistake to believe that these professors represented the considered opinion of the best minds, while the mathematical practitioners were oddball underdogs whose pioneering success eventually proved undeniable to the surprise of everyone. The mathematical practitioners stood for simple common sense, not renegade iconoclasm. They practiced the same common sense that their peers had in antiquity, with much the same results. The university professors, meanwhile, should not be mistaken for a neutral representation of the state of human knowledge at the time. Rather, they formed one particular philosophical sect which retained its domination of the universities not because of the preeminence of its teachings but because of the conservative appointment practices and obsequiousness of academics.
So that’s my take on the role of practical mathematics in the scientific revolution.
Now let’s turn to another issue, a more philosophical one: instrumentalism versus realism.
A standard view is that “the Scientific Revolution saw the replacement of a predominantly instrumentalist attitude to mathematical analysis with a more realist outlook.” Instrumentalism means the following; I’m quoting Simplicius the ancient commentator:
“An explanation which conforms to the facts does not imply that the hypotheses are real and exist. [Astronomers] have been unable to establish in what sense, exactly, the consequences entailed by these arrangements are merely fictive and not real at all. So they are satisfied to assert that it is possible, by means of circular and uniform movements, always in the same direction, to save the apparent movements of the wandering stars.”
Instrumentalism, as opposed to realism, was supposedly the accepted philosophy of science among “the Greeks,” according to many historians. Here’s what Pierre Duhem had to say about it for example:
“[Ancient Greek astronomers] balked at the idea that the eccentrics and epicycles are bodies, really up there on the vaults of the heavens. For the Greeks they were simply geometrical fictions requisite to the subjection of celestial phenomena to calculation. If these calculations are in accord with the results of observation, if the ‘hypotheses’ succeed in ‘saving the phenomena’, the astronomer’s problem is solved.”
“An astronomer who understands the true purpose of science, as defined by men like Posidonius, Ptolemy, Proclus, and Simplicius, … would not require the hypotheses supporting his system to be true, that is, in conformity with things. For him it will be enough if the results of calculation agree with the results of observation—if appearances are saved.”
That’s Duhem, in the early 20th century. But plenty of modern historians agree as well. Here are some examples, I quote:
“The Greek geometer in formulating his astronomical theories does not make any statements about physical nature at all. His theories are purely geometrical fictions. That means that to save the appearances became a purely mathematical task, it was an exercise in geometry, no more, but, of course, also no less.”
Galileo, by contrast, brought “a radically new mode of realist-mathematical nature knowledge.”
In other words:
“Galileo endorsed a view that was [contrary to] that of the Greeks but was also much more creative … It is a crippling restriction to hold that no theory about reality can be in mathematical form; the Renaissance rejected this restriction, holding that it was a worthwhile enterprise to search for mathematical theories which also—by metaphysical criteria—could be supposed ‘real’. … The most eloquent and full defence of this process was given by Galileo.”
Hence the Scientific Revolution owes much to “the novel quality of realism that the abstract-mathematical mode of nature-knowledge acquired in Galileo’s hands.”
All of that are quotations from mainstream historical scholarship. I of course disagree with them, as you might imagine.
In reality, no mathematically competent Greek author ever advocated instrumentalism. The notion that “the Greeks” were instrumentalists relies exclusively on passages by philosophical commentators. The notion that Ptolemy believed his planetary models were “fictional combinations of circles which could never exist in celestial reality” is demonstrably false.
First of all Ptolemy opens his big book with physical arguments for why the earth is in the center of the universe. This is a blatantly realist justification for this aspect of his astronomical models.
Furthermore, Ptolemy has a detailed discussion of the order and distances of the planets that obviously assumes that the planetary models, epicycles and all, are physically real. “The distances of the … planets may be determined without difficulty from the nesting of the spheres, where the least distance of a sphere is considered equal to the greatest distance of a sphere below it.” That is to say, according to Ptolemy’s epicyclic planetary models, each planet sways back and forth between a nearest and a furthest distance from the earth. The “sphere” of each planet must be just thick enough to contain these motions. Ptolemy assumes that “there is no space between the greatest and least distances [of adjacent spheres],” which “is most plausible, for it is not conceivable that there be in Nature a vacuum, or any meaningless and useless thing.”
Clearly this is based on taking planetary models to be very real indeed, and not at all mathematical fictions invented for calculation. Nor was Ptolemy an exception in his realism. His colleague Geminos “was a thoughtful realist” too, as the translators of his surviving astronomical work have observed.
Hipparchus too evidently chose models for planetary motion on realist grounds. His works are lost, but we know that he proved the mathematical equivalence of epicyclic and equant motion. In other words, he showed that two different geometrical models of planetary motion are observationally equivalent; they lead to the exact same visual impressions seen from earth, but they are brought about by different mechanisms. How should one choose between the two models in such a case? If Hipparchus was an instrumentalist, he wouldn’t care one way or the other, or he would just pick whichever was more mathematically convenient. But if he was a realist he would be interested in which model could more plausibly correspond to actual physical reality. So what did he do? Here is what Theon says: “Hipparchus, convinced that this is how the phenomena are brought about, adopted the epicyclic hypothesis as his own and says that it is likely that all the heavenly bodies are uniformly placed with respect to the center of the world and that they are united to it in a similar way.” So Hipparchus decided between equivalent models based on physical plausibility. This is quite clearly a realist argument.
Historians have brought up other “evidence” that “the Greeks” were instrumentalists. One thing they point to is the alleged compartmentalisation of Greek science. I quote a modern historian:
“Phenomena [such as] consonance, light, planetary trajectories and the two states of equilibrium [i.e., statics and hydrostatics] are investigated separately. There is no search for interconnections, let alone for an overarching unity.”
This attitude would indeed make sense if mathematical science was just instrumental computation tools with no genuine anchoring in reality. The only problem is that the claim is false. Greek science is in fact full of interconnections, just as one would expect if they were committed realists. Ptolemy uses mechanics to justify geocentrism; Archimedean hydrostatics explains shapes of planets and “casts light on the earth’s geological past”; Archimedes used statical principles to compute areas in geometry. Ptolemy applies “consonance” (that is, musical theory) to “the human soul, the ecliptic, zodiac, fixed stars, and planets,” as he says in his book on astrology. Ptolemy also applies the law of refraction of optics to atmospheric refraction, noting its importance for astronomical observations.
In Galileo’s time, the same pattern prevails: mathematically competent people are unabashed realists, while philosophers and theologians often find instrumentalism more appealing for reasons that have nothing to do with science. Copernicus’s book, for example, is unequivocally realist. Spineless philosophers and theologians could not accept this. One even resorted to the ugly trick of inserting an unsigned foreword in the book without Copernicus’s authorisation, in which they espoused instrumentalism. Here’s what is says:
“It is the job of the astronomer to use painstaking and skilled observation in gathering together the history of the celestial movements, and then—since he cannot by any line of reasoning reach the true causes of these movements—to think up or construct whatever causes or hypotheses he pleases such that, by the assumption of these causes, those same movements can be calculated from the principles of geometry for the past and for the future too. … It is not necessary that these hypotheses should be true. … It is enough if they provide a calculus which fits the observations.”
This foreword was left unsigned so that it was easy to assume that it was written by Copernicus himself. This surely fooled no one who actually read the book, with all its blatant realism. Giordano Bruno, for one, thought “there can be no question that Copernicus believed in this motion [of the earth],” and hence concluded that the timid foreword must have been written “by I know not what ignorant and presumptuous ass.” That’s Bruno’s opinion, a early reader of Copernicus. Other mathematical readers presumably felt the same way. But then again the mathematically incompetent people whom the instrumentalist foreword was designed to appease could not read the book anyway.
In medieval and renaissance philosophical texts it is not hard to find many assertions to the effect that “real astronomy is nonexistent” and what passes for astronomy “is merely something suitable for computing the entries in astronomical almanacs.” There were many instrumentalists at the time, to be sure, but the challenge is to find a single serious mathematical astronomer among them. They were exclusively theologians and philosophers.
All historians nowadays recognise that “Copernicus clearly believed in the physical reality of his astronomical system,” but their inference that he “thus broke down the traditional disciplinary boundary between astronomy (a branch of mixed mathematics) and physics (or natural philosophy)” is dubious. This was “the traditional” view only in a very limited sense. It was traditional among the particular sect of Aristotelians that occupied the universities, but outside this narrow clique it had no credibility or standing whatsoever. Among mathematicians, Copernicus’s view was exactly the traditional one.
All mathematically competent people continued in the same vein, long before Galileo entered the scene. Already in the 16th century, “Tycho and Rothman, Maestlin, and even Ursus openly deploy a wide range of physical arguments in debating the issue between the rival world-systems.” Kepler puts the matter very clearly:
“One who predicts as accurately as possible the movements and positions of the stars performs the task of the astronomers well. But one who, in addition to this, also employs true opinions about the form of the universe performs it better and is held worthy of greater praise. The former, indeed, draws conclusions that are true as far as what is observed is concerned; the latter not only does justice in his conclusions to what is seen, but also in drawing conclusions embracing the inmost form of nature.”
As Kepler notes, this was all obviously well-known and accepted since antiquity, for “to predict the motions of the planets Ptolemy did not have to consider the order of the planetary spheres, and yet he certainly did so diligently.”
So, in conclusions, mathematicians were always realists. Galileo had nothing new to contribute on that matter. So we have refuted that as well as one of the possible Galilean innovations that caused the scientific revolution.
Here’s another of the big themes in the scientific revolution: the “mechanical philosophy.”
Some say that “the mechanization of the world-picture” was the defining ingredient of “the transition from ancient to classical science.” A paradigm conception at the heart of the new science was that of the world as a machine: a “clockwork universe” in which everything is caused by bodies pushing one another according to basic mechanical laws, as opposed to a world governed by teleological purpose, divine will and intervention, anthropomorphised desires and sympathies ascribed to physical objects, or other supernatural forces. Galileo was supposedly a pioneer in how he always stuck to the right side in this divide. Here is one historian arguing as much:
“Galileo possessed in a high degree one special faculty. That is the faculty of thinking correctly about physical problems as such, and not confusing them with either mathematical or philosophical problems. It is a faculty rare enough still, but much more frequently encountered today than it was in Galileo’s time, if only because nowadays we all cope with mechanical devices from childhood on.”
Of course, this “special faculty” is precisely what led Galileo to reject as occult the correct explanation of the tides and propose his own embarrassing nonstarter of a tidal theory based on an analogy with “mechanical devices,” as we have discussed before. But let’s put that aside.
There is nothing modern about the mechanical philosophy. “*We* all cope with mechanical devices from childhood on,” the quote says, but so did the Greeks. They built automata such as entirely mechanical puppet-theatres, self-opening temple doors, a coin-operated holy water dispenser, and so on. Pappus notes that “the science of mechanics” has many applications “of practical utility,” including machines for lifting weights, warfare machines such as catapults, water-lifting machines, and “marvellous devices” using “ropes and cables to simulate the motions of living things.”
Clearly, then, “Ancient Greek mechanics offered working artifacts complex enough to suggest that organisms, the cosmos as a whole, or we ourselves, might ‘work like that’.” Thus we read in ancient sources that “the universe is like a single mechanism” governed by simple and deterministic laws that ultimately lead to “all the varieties of tragic and comedic and other interactions of human affairs.” This line of reasoning soon lead to a secularisation of science. “Bit by bit, Zeus was relieved of thunderbolt duty, Poseidon of earthquakes, Apollo of epidemic disease, Hera of births, and the rest of the pantheon of gods were pensioned off” in the same manner.
Mechanical explanations are widespread in Greek science. The Aristotelian Mechanics uses the law of lever to explain “why rowers who are in the middle of the ship move the ship the most,” and “how it is that dentists extract teeth more easily by a tooth-extractor [or forceps] than with the bare hand only.” Greek scientists explained perfectly clearly that sound is a “wave of air in motion,” comparable to the rings forming on a pond when when one throws in a stone. Atomism—a widely espoused conception of the world in Greek antiquity—is of course in effect a plan to “make material principles the basis of all reality.”
Greek astronomy went hand in hand with mechanical planetaria that directly reproduced a scale model of planetary motion. And not just basic toy models, but “complex and scientifically ambitious instruments” that could generate all heavenly motions mechanically from a single generating motion (the turn of a crank, as it were).
The possibility that even biological phenomena worked on the same principle immediately suggested itself and was eagerly pursued. Here’s what Galen says, the ancient physician:
“Just as people who imitate the revolutions of the wandering stars by means of certain instruments instill a principle of motion in them and then go away, while [the devices] operate just as if the craftsman was there and overseeing them in everything, I think in the same way each of the parts in the body operates by some succession and reception of motion from the first principle to every part, needing no overseer.”
Indeed, ancient medical research put this vision into practice. “The use of what we should call mechanical ideas to explain organic processes”—such as digestion and other physiological functions—is “the most prominent feature” of the work of Erasistratus in medicine, who also tested his ideas experimentally.
In conclusion, then, the world did not need Galileo to tell them about the mechanical philosophy, since it had been widely regarded as common sense already in antiquity.
The scientific revolution did not come about by any innovative or groundbreaking insights of Galileo. It came about by simply listening to what the mathematicians had been saying for thousands of years.
from Intellectual Mathematics







 }
}